Jérôme Coville

Research interests

My research focuses on the design, analysis and simulation of population dynamics models involving long-range interactions, also called non-local models. Depending on the study context (ecology, epidemiology, demo-genetics), these long-range interactions result from the modeling of different biological/ecological processes such as long-distance dispersal processes, mutation processes or even competition processes (inter/intraspecific). Thus most of my work focuses :

• i) on the analysis of integro-differential models integrating these long-range processes,
• ii) on the way to simulate such type of models
• iii) on their use in order to deepen our knowledge on dynamic phenomena in conservation ecology, on the phenomena of biological invasion, but also on issues of adaptation to global change.

Focus on some recent studies

Spectral theory of positive nonlocal operators:

Recently, I have been interested in extending the concept of generalised principal eigenvalue for positive nonlocal operators, concept and definition introduced to describe ellliptic operators. Namely, I have mainly focus my effort on finding useful variational definitions as surogate for the principal eigenvalue of a positive operator and studying their main properties. The existence of a principal eigenfunction is one of the main objectif of the criteria I'm developping. Here is an example where these quantity can only be associated to a measure comporting a singular part.

Propagation Phenomena in complex domain:

The landscape is rarely homogeneous and often contains multiple structures (roads, forests, fields, lake, ... ) that can affect the propagation of individuals. With F. Hamel, E. Valdinoci and J. Brasseur, we are interested in how simple geometric element in a domain can affect propagation phenomena modelled by nonlocal diffusion. We especially study the influence of the distance considered to build the nonlocal diffusion.

On the left, a simulation of the propagation in a 3d domain, on the right simulations of the effect of the chosen distance (geodesic vs euclidean).

Acceleration phenomena in reaction dispersal equation :

Long range dispersal process may induced accelerated invasion process, a characterisation of speed of invasion is then of great importance as well of the determinant of such acceleration process. In particular, identifying key factors such as the shape of the kernel and or the growth of a population is of prime interest in the perspective of set up efficient control measures, for example in a epidemiological context. In a series of works, in collaboration with M. Alfaro, E. Bouin, C. Gui, G. Legendre, M. Zhao, and X. Zhang we have tried to understand the mechanisms that trigger such acceleration processes and gives some quantitative estimates on the speed of the propagation with respect to the main properties of the dispersal kernel and the growth rate of population at low density.

On the left typical kernel considered, In the middle and at the right, two descriptions of the speed obtained in terms of the decay of the kernel and the strength of the growth at low density. At the center it describe situation where the growth is a power like function whereas at the right the growth is in beetween linear and a powerlike function.

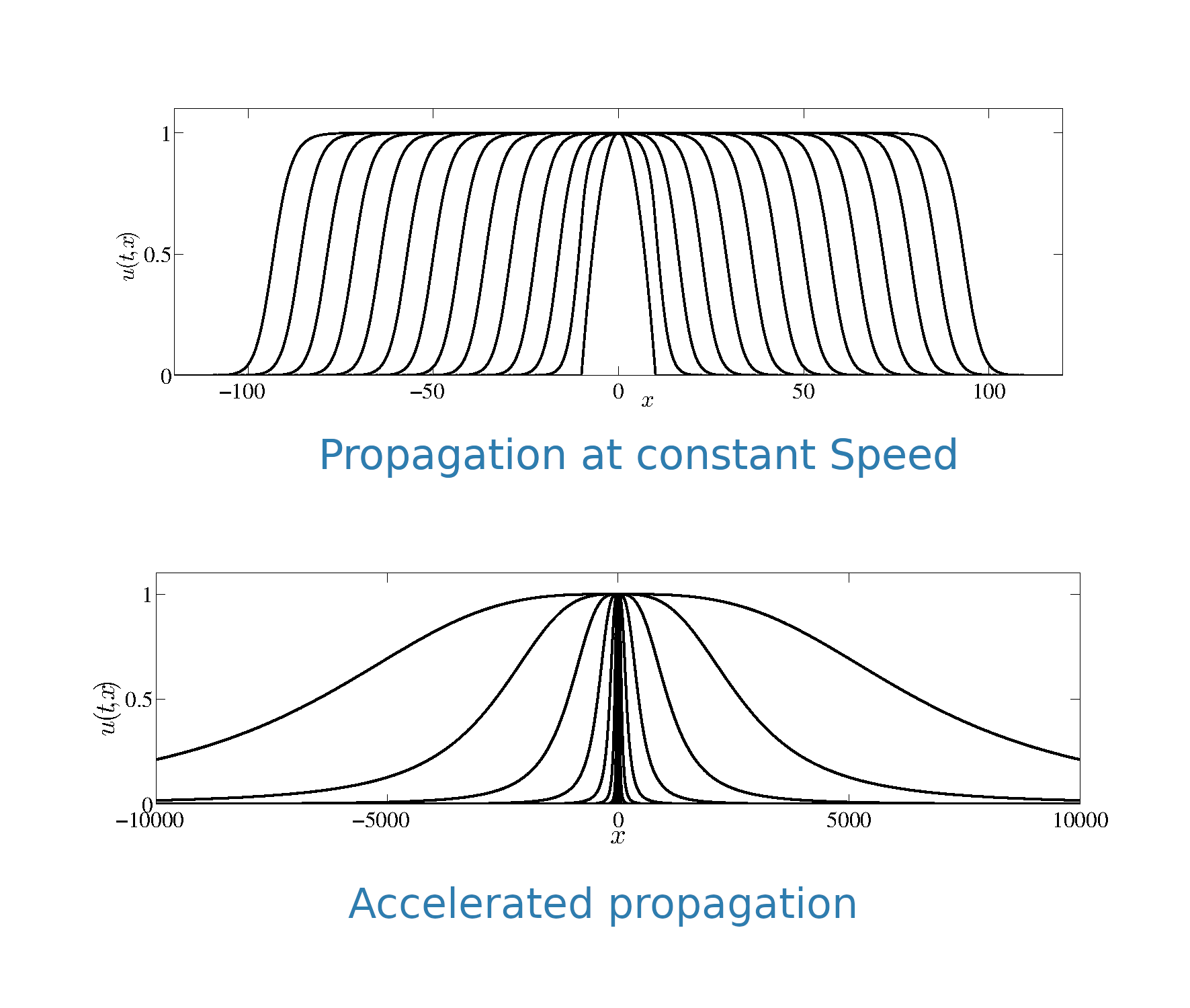
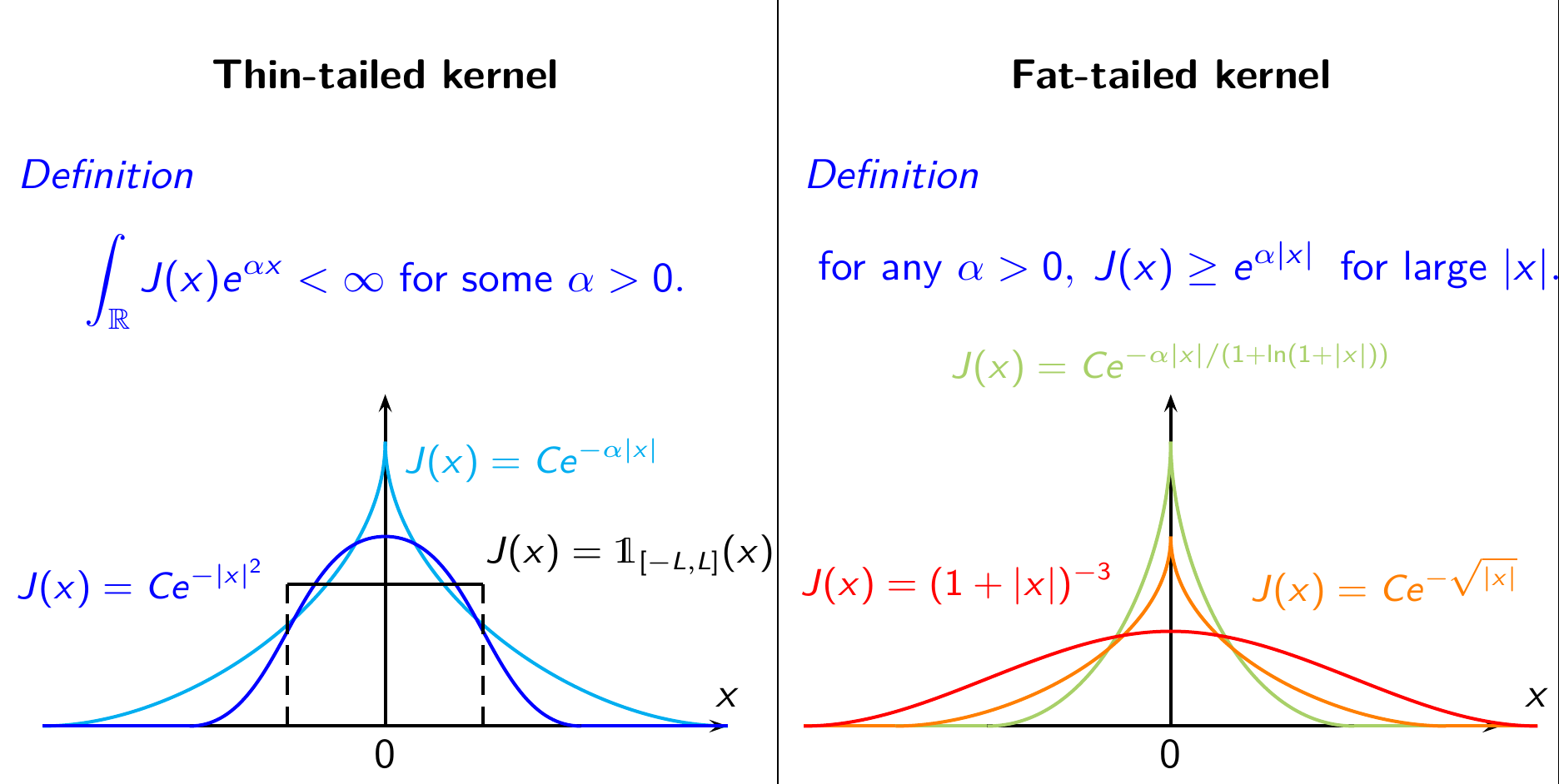
Habilitation à Diriger les Recherches (2015):

Contribution à l'étude d'équations non locales en dynamique des populations 
Mémoire d'HDR, soutenue le 16 Novembre 2015 https://tel.archives-ouvertes.fr/tel-01238013/document.

Phd Thesis (2003):

Equation de réaction diffusion non-locale
Thèse de doctorat, soutenue le 18 Novembre 2003. https://tel.archives-ouvertes.fr/tel-00004313/document

New preprint

Bouin, E., Coville, J.  (2025). Travelling waves in non-local reaction-dispersion equations with diffuse Levy measures. https://arxiv.org/abs/2503.20381

Alvarez-Latuz, C. Bayen, T. & Coville, J. (2025). Global stability of perturbed chemostat systems. https://arxiv.org/abs/2501.08011

Bouin, E., Coville, J.,  Zhang, X.  (2024). Acceleration or finite speed propagation in integro-differential equations with logarithmic Allee effect. https://arxiv.org/abs/2412.06505  

Ayala, M., Coville, J., & Soubeyrand, S. (2024). Group Dispersal Modelling revisited. https://arxiv.org/abs/2405.08384

Bouin, E., Coville, J., & Legendre, G. (2021). Acceleration in integro-differential combustion equations. arXiv preprint arXiv:2105.09946. https://arxiv.org/abs/2105.09946

Preprint: 

Coville, J., & Fabre, F. (2013). Convergence to the equilibrium in a Lotka-Volterra ODE competition system with mutations. arXiv preprint arXiv:1301.6237. http://fr.arxiv.org/abs/1301.6237

Coville, J. (2013). Convergence to equilibrium for positive solutions of some mutation-selection model. arXiv preprint arXiv:1308.6471.http://arxiv.org/abs/1308.6471

Articles publiés:

49. Bouin, E., Coville, J., & Legendre, G. (2025). Sharp exponent of acceleration in general nonlocal equations with a weak Allee effect, to appear in Annales de la Faculté de Sciences de Toulouse, https://hal.archives-ouvertes.fr/hal-03452141v1

48. Ayala, M., Coville, J., & Forien, R. (2024). A measure-valued stochastic model for vector-borne viruses, to appear in ALEA, https://arxiv.org/abs/2211.04563

47. Bouin, E., Coville, J.,  Zhang, X.  (2024)  Precise rates of propagation in reaction–diffusion equations with
logarithmic Allee effect,  Nonlinear Analysis, 245,   https://arxiv.org/abs/2312.09614, https://doi.org/10.1016/j.na.2024.113557

46.Saubin, M., Coville, J.,  Xhaard, C., Frey, P., Soubeyrand, S., Halkett, F., Fabre, F. (2024) Inferring invasion determinants with mechanistic models and multitype sample,   Peer Community Journal, Volume 4, article  no. e9. doi : 10.24072/pcjournal.356. https://peercommunityjournal.org/articles/10.24072/pcjournal.356/  

45. Bouin, E., Coville, J., & Legendre, G. (2023). A simple flattening lower bound for solutions to some linear integrodifferential equations.  Z. Angew. Math. Phys. 74:234  https://arxiv.org/abs/2303.00101 https://doi.org/10.1007/s00033-023-02118-2 

44. Gabriel, E., Rodríguez-Cortes, F. J., Coville, J., Mateu, J., & Chadoeuf, J. (2023). Mapping the intensity function of a non-stationary point process in unobserved areas.  Stoc. Env. Res. & Ris. Ass., 37(1), 327-343. https://arxiv.org/abs/2111.14403 , https://doi.org/10.1007/s00477-022-02304-0

43. Bayen,T., Coville, J., & Mairet, F. (2023). Stabilization of the chemostat system with mutations and application to microbial production.  Optim Control Appl Meth.; 44(6): 3342–3360.  https://hal.archives-ouvertes.fr/hal-03767646v1 https://doi.org/10.1002/oca.3041

42. Bayen, T., Cazenave-Lacroutz, H.,  Coville, J. & Mairet, F. (2022). Optimal control of microbial production in the chemostat  IFAC 55,16, 208-213 . https://doi.org/10.1016/j.ifacol.2022.09.025

41. Bayen, T., Cazenave-Lacroutz, H., & Coville, J. (2022). Stability of the chemostat system including a linear coupling between species.  Discrete and Continuous Dynamical Systems-B, 28(3), 2104-2129. https://arxiv.org/abs/2110.09582,  https://doi.org/10.3934/dcdsb.2022160

40. Brasseur, J., & Coville, J. (2021). Propagation phenomena with nonlocal diffusion in presence of an obstacle. Journal of Dynamics and Differential Equations, 1-65. https://arxiv.org/abs/2004.13360

39. Martin, O., Fernandez-Diclo, Y., Coville, J., & Soubeyrand, S. (2021). Equilibrium and sensitivity analysis of a spatio-temporal host-vector epidemic model. Nonlinear Analysis: Real World Applications, 57, 103194. https://arxiv.org/abs/2002.07542

38. Fabre, F., Coville, J., & Cunniffe, N. J. (2021). Optimising reactive disease management using spatially explicit models at the landscape scale. In Plant Diseases and Food Security in the 21st Century (pp. 47-72). Springer, Cham. https://arxiv.org/abs/1911.12131

37. Coville, J., Gui, C., & Zhao, M. (2021). Propagation acceleration in reaction diffusion equations with anomalous diffusions. Nonlinearity, 34(3), 1544.https://arxiv.org/abs/2003.05446

36. Coville, J. (2021). Can a population survive in a shifting environment using non-local dispersion?. Nonlinear Analysis, 212, 112416.https://arxiv.org/abs/2012.09441

35. Coville, J., & Hamel, F. (2020). On generalized principal eigenvalues of nonlocal operators witha drift. Nonlinear Analysis, 193, 111569. https://arxiv.org/abs/1812.11412

34. Brasseur, J., & Coville, J. (2020). A counterexample to the Liouville property of some nonlocal problems. In Annales de l'Institut Henri Poincaré C, Analyse non linéaire (Vol. 37, No. 3, pp. 549-579). Elsevier Masson. https://arxiv.org/abs/1804.07485

33. Coville, J. (2020). A Note on Liouville type results for a fractional obstacle problem. In 2018 MATRIX Annals (pp. 215-228). Springer, Cham. https://arxiv.org/abs/1903.00341

32. Brasseur, J., Coville, J., Hamel, F., & Valdinoci, E. (2019). Liouville type results for a nonlocal obstacle problem. Proceedings of the London Mathematical Society, 119(2), 291-328. https://arxiv.org/abs/1712.09877

31. Alfaro, M., & Coville, J. (2017). Propagation phenomena in monostable integro-differential equations: acceleration or not?. Journal of Differential Equations, 263(9), 5727-5758.https://arxiv.org/abs/1610.05908

30. Li, F., Coville, J., & Wang, X. (2017). On eigenvalue problems arising from nonlocal diffusion models. Discrete & Continuous Dynamical Systems, 37(2), 879.

29. Gabriel, E., Coville, J., & Chadoeuf, J. (2017). Estimating the intensity function of spatial point processes outside the observation window. Spatial Statistics, 22, 225-239.

28. Bonnefon, O., Coville, J., & Legendre, G. (2017). Concentration phenomen in some non-local equation. Discrete and Continuous Dynamical Systems-Series B, 22(3), 763-781..   http://arxiv.org/pdf/1510.01971v1

27. Berestycki, H., Coville, J., & Vo, H. H. (2016). On the definition and the properties of the principal eigenvalue of some nonlocal operators. Journal of Functional Analysis, 271(10), 2701-2751.https://arxiv.org/abs/1512.06529

26. Berestycki, H., Coville, J., & Vo, H. H. (2016). Persistence criteria for populations with non-local dispersion. Journal of mathematical biology, 72(7), 1693-1745. http://arxiv.org/pdf/1406.6346v2

25. Coville, J. (2015). Nonlocal refuge model with a partial control. Discrete and Continuous Dynamical Systems-Series A, 35(4), 1421-1446.   http://arxiv.org/abs/1305.7122

24. Bonnefon, O., Coville, J., Garnier, J., Hamel, F., & Roques, L. (2014). The spatio-temporal dynamics of neutral genetic diversity. Ecological Complexity, 20, 282-292.

23. Bonnefon, O., Coville, J., Garnier, J., & Roques, L. (2014). Inside dynamics of solutions of integro-differential equations. Discrete & Continuous Dynamical Systems-B, 19(10), 3057-3085. https://doi.org/10.3934/dcdsb.2014.19.3057

22. Alfaro, M., Coville, J., & Raoul, G. (2014). Bistable travelling waves for nonlocal reaction diffusion equations. Discrete and Continuous Dynamical Systems-Series A, 34(5), 1775-1791.    http://fr.arxiv.org/abs/1303.3554

21. Coville, J. (2013). Singular measure as principal eigenfunction of some nonlocal operators. Applied Mathematics Letters, 26(8), 831-835. http://fr.arxiv.org/abs/1302.0949

20. Alfaro, M., Coville, J., & Raoul, G. (2013). Travelling waves in a nonlocal reaction-diffusion equation as a model for a population structured by a space variable and a phenotypic trait. Communications in Partial Differential Equations, 38(12), 2126-2154.  http://fr.arxiv.org/abs/1211.3228

19. Coville, J., Dávila, J., & Martínez, S. (2013). Pulsating fronts for nonlocal dispersion and KPP nonlinearity. In Annales de l'Institut Henri Poincaré C, Analyse non linéaire (Vol. 30, No. 2, pp. 179-223). Elsevier Masson. http://fr.arxiv.org/abs/1302.1053

18. Alfaro, M., & Coville, J. (2012). Rapid traveling waves in the nonlocal Fisher equation connect two unstable states. Applied Mathematics Letters, 25(12), 2095-2099.http://fr.arxiv.org/abs/1205.2349

17. Fabre, F., Montarry, J., Coville, J., Senoussi, R., Simon, V., & Moury, B. (2012). Modelling the evolutionary dynamics of viruses within their hosts: a case study using high-throughput sequencing. PLoS Pathogens, 8(4), e1002654. http://www.plospathogens.org/article/info%3Adoi%2F10.1371%2Fjournal.ppat.1002654

16. Coville, J. (2012). Harnack type inequality for positive solution of some integral equation. Annali di Matematica Pura ed Applicata, 191(3), 503-528. http://fr.arxiv.org/abs/1302.1677

15. Soubeyrand, S., Roques, L., Coville, J., & Fayard, J. (2011). Patchy patterns due to group dispersal. Journal of Theoretical Biology, 271(1), 87-99.

14. Coville, J., & Dávila, J. (2011). Existence of radial stationary solutions for a system in combustion theory. Discrete & Continuous Dynamical Systems-B, 16(3), 739. http://fr.arxiv.org/abs/1106.5597

13. Coville, J., Dirr, N. P., & Luckhaus, S. (2010). Non-existence of positive stationary solutions for a class of semi-linear PDEs with random coefficients. Networks and Heterogeneous Media, 5(4), 745-763. http://fr.arxiv.org/abs/1106.5138

12. Coville, J. (2010). On a simple criterion for the existence of a principal eigenfunction of some nonlocal operators. Journal of Differential Equations, 249(11), 2921-2953, http://fr.arxiv.org/abs/1106.5137

11. Coville, J. (2008). Remarks on the strong maximum principle for nonlocal operators. Electronic Journal of Differential Equations (EJDE)[electronic only], 2008, Paper-No. http://ejde.math.txstate.edu/Volumes/2008/66/abstr.html

 10. Coville, J., Dávila, J., & Martínez, S. (2008). Nonlocal anisotropic dispersal with monostable nonlinearity. Journal of Differential Equations, 244(12), 3080-3118.. http://fr.arxiv.org/abs/1106.4531

9. Coville, J., Dávila, J., & Martínez, S. (2008). Existence and uniqueness of solutions to a nonlocal equation with monostable nonlinearity. SIAM Journal on Mathematical Analysis, 39(5), 1693-1709.http://fr.arxiv.org/abs/1106.5135

8. Coville, J. (2007). Travelling fronts in asymmetric nonlocal reaction diffusion equations: the bistable and ignition cases. Hal-00696208, http://hal.archives-ouvertes.fr/index.php?action_todo=search&view_this_doc=hal-00696208&version=1&halsid=ngfvlf0sblu5tbe17mcp0e5qi4

7. Coville, J. (2007). Maximum principles, sliding techniques and applications to nonlocal equations. Electronic Journal of Differential Equations (EJDE)[electronic only], 2007, Paper-No. http://ejde.math.txstate.edu/Volumes/2007/68/abstr.html

6. Cortazar, C., Coville, J., Elgueta, M., & Martinez, S. (2007). A nonlocal inhomogeneous dispersal process. Journal of Differential Equations, 241(2), 332-358.

5. Coville, J., & Dupaigne, L. (2007). On a non-local equation arising in population dynamics. Proceedings of the Royal Society of Edinburgh Section A: Mathematics, 137(4), 727-755.

4. Coville, J. (2006). On uniqueness and monotonicity of solutions of non-local reaction diffusion equation. Annali di Matematica Pura ed Applicata, 185(3), 461-485.

3. Coville, J., & Dupaigne, L. (2005). Propagation speed of travelling fronts in non local reaction–diffusion equations. Nonlinear Analysis: Theory, Methods & Applications, 60(5), 797-819.

2. Coville, J., & Dupaigne, L. (2003). Travelling fronts in integrodifferential equations. Comptes Rendus Mathematique, 337(1), 25-30.

1. Coville, J. (2003). Monotonicity in integrodifferential equations. Comptes Rendus Mathematique, 337(7), 445-450.